When Guitar World first approached me about writing a monthly column, I thought they were kidding. I mean, how can a hack like me actually sustain a series on anything?
Seriously, though, as the sole guitar player in Linkin Park, I have the freedom and responsibility to fulfill all sorts of roles. For instance, I might play an orchestral harmonic loop in one part of a song and then a power chord rhythm figure right after that. In other words, I have to have versatility-something that comes from listening to and studying many styles of music.

I'm a huge fan of everything from emo to hip- hop to techno to hardcore. I didn't get into each style at the same time, though. Rather, I went through many different musical phases, as I'm sure most of you have. When I started playing in bands, my friends and I realized that we could create a unique sound by combining our love for disparate styles into one giant musical melting pot. Starting in 1996, as Xero, we began to experiment by mixing beats with guitars, and combining singing with rapping. Since we didn't have a record deal or any real way to get our music to the masses, we focused on our songwriting and the evolution of our hybrid sound. Now that we're acquainted, let's skip the foreplay and cover some basic musical facts that all musicians should know. Everything that we hear reaches our ears via sound waves. Waves that vibrate faster are said to have a higher frequency and, consequently, a higher pitch. The spectrum of sound waves is divided up into certain arbitrary points or locations. These designated points represent pitches. The distance, or interval, from any pitch to one that's exactly twice its frequency on the spectrum is called an octave. This particular mathematical relation- ship between the two pitches is an important one, because we use it to organize our whole sys- tem of harmony. It's also important because we perceive the two pitches as sounding almost the same. The only difference, as far as our ears and brains are concerned, is that the pitch that's twice the frequency of the other one sounds higher. For example, the open E note on the first string and the E note at the 12th fret on that same string are said to be an octave apart. Notice how the two E notes sort of sound the same, yet different.

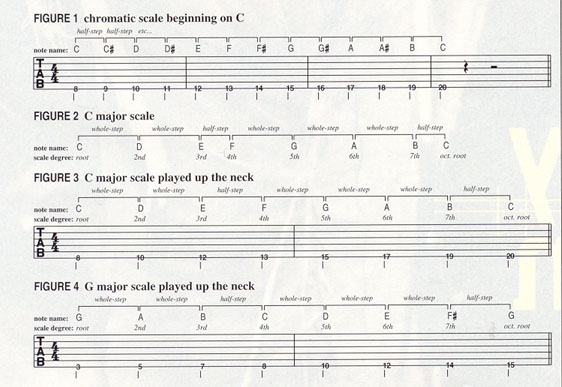
An octave is divided into 12 half steps, or semitones. On the guitar, a half step is two notes that are right next to each other (one fret apart) on the same string. The complete set of 12 half steps that fall, within an octave comprises what's known as the chromatic scale. If you were to start on the note C, the scale would be spelled as follows: C C# D D# E F F# G G# A A# B C. The last note is an octave higher than the starting note, C. Let's check this out on the guitar. Start at the eighth fret on the sixth (low E) string. That note is C. Now move up the string, one fret (half step) at a time, until you get to the 20th fret, which is the C note an octave higher than the C we started on at the eighth fret. This is illustrated in FIGURE 1.

What we are accustomed to hearing in West- ern music, though, is not the chromatic scale but a specific sequence of half steps and whole steps (a whole step is equal to two frets on the guitar) that form the major scale. We can see an example of this by looking at the C-major scale, the only major scale that contains no sharp (#) notes. The C-major scale is spelled C DE F GAB C. This scale conforms to the standard major-scale interval pattern, which is: whole step, whole step, half step, whole step, whole step, whole step, half step (FIGURE 2).

Once again, let's check this out on your guitar. Start on the C note at the eighth fret on the sixth string, and then move up this string by the designated interval pattern. Remember, a whole step is a two-fret jump, while a half step is only one fret. If you sound out these intervals correctly, you'll hear the C-major scale(FIGURE 3).

The cool thing about this interval pattern is that it works at any starting point on any string, something that will enable you to teach your- self how to playa major scale beginning on any note. For example, FIGURE 4 depicts the major scale starting on the note G, at the third fret on the low E string. You'll notice the interval pat- tern is exactly the same (whole, whole, half, whole, whole, whole, half), but this time we see that one of the note names contains a sharp (#). We have to play F# as opposed to F because the seventh note of the scale needs to be a whole step higher than the sixth note, which in this case is E. You'll also see that F# is the right distance from the next note in the scale, G, which is a half step above it.

As a self-teaching exercise, practice using the "whole step, whole step, half step, whole step, whole step, whole step, half step" formula to construct major scales up the neck on every string, starting on the note D. Then do the same thing starting on A and E. Be sure to do this, because you're going to need the practice in order to tackle next month's lesson. Hey, I'm taking my time out from the debauchery of touring life to work on these columns, so I expect you to make time for this, too. Peace out.